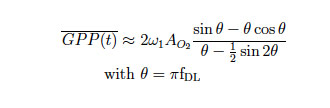Disclaimer:
The authors are solely responsible for the content of this report. Material included herein does not represent the opinion of the European Community, and the European Community is not responsible for any use that might be made of it.
Back to overview reports
As is clear from this table, comparison for biogeochemistry for all four TIDE estuaries can only be applied on a limited set of parameters. Furthermore, not all zones are equally represented in every estuary (table 1). Comparison and analyses are performed whenever at least two estuaries have data for the parameters of interest for the research question addressed (see ‘1 Introduction’ ).
For summary statistics: number (n), mean, median, standard error, minimum and maximum of all variables
Table 2 Summary overview of biogeochemical data availability per estuary (x* very few data points, x deduced data, x(!) chlorophyll extract for the Humber estuary, chlorophyll a data not available)
Furthermore, light climate is discussed by approximation of euphotic depth and mixing depth. Euphotic depth is calculated from suspended particulate matter concentrations with the following formulas according to Holzhauer et al. 2011:
Separate principal component analyses (PCA, Dolédec and Chessel 1987; Thioulouse et al. 2004) were performed for each estuary. Between-class analyses revealed the structuring influence for year, zone and season. The assessment involves a Monte-Carlo procedure based on 999 random permutations of the lines of the data table returning 999 simulated values of inertia, plus the observed one; the significance of the effect is then tested by the proportion of simulated values greater than the observed one (Heo and Gabriel 1999).
The partial triadic analysis (PTA, Thioulouse and Chessel 1987; Blanc et al. 1998; Thioulouse et al. 2004) is a sophisticated within-group analysis whereby the influence of a given factor (e.g. salinity zone or season here) is masked. In this multi-table approach, a set of k tables are seen as k clouds of points between which structural similarities are investigated. Its application here is especially relevant since the data form a cube defined by fully matched tables (e.g. same seasons × same descriptors × same zones). The columns of each table are initially centered (value minus mean value) so that all the clouds of point have a common geometric origin, and by this way, the considered factor is masked and the amplitude of each cloud of point is conserved. The procedure comprises two main steps:
(1) The construction of a correlation matrix summarizing the strength of multidimensional similarity between the tables; this step is called “interstructure”, and consists in identifying the most similar / deviant patterns by mean of a correlation circle; the statistics used is the Rv coefficient correlation (Robert and Escoufier 1976), and its significance is tested based on 999 random permutations of the lines of the tables.
(2) The construction of a system of axes encompassing the common processes between the tables as a common model, called “compromise”. As a second sub-step, the projection of the lines and columns of the different tables on the axes provides a mechanistic understanding of the similarities / deviations highlighted in the so-called “intrastructure”.
To have a more overall view, this gain or loss has also been multiplied with freshwater discharge, hence total gain or loss can be compared between estuaries. This has been performed for the whole estuarine gradient and per zone. Filter efficiencies have been calculated in percentage as the gain or loss according to the input concentration. The input concentrations per zone are defined as the most upstream concentration of that zone.
Residence time can reach up to about maximum 40 days in the freshwater zone (Elbe) and about maximum 50 days in the polyhaline zone (Scheldt) in summer. Thus, seasonal gain or loss can be considered. However, a yearly average can be considered more accurate, since transient effects are attenuated in this way.
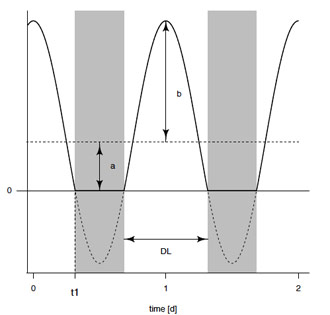
A truncated sinusoid (fig. 8) relation is applied between the time average and Fourier amplitude, which is function of the fraction of daylight. This transformation assumes a constant daylight fraction. Therefore, only time series of 14 days are considered at once.
The method is less applicable when the estuarine system is stratified, shallow, subject to short residence times, experiencing strong air-water exchange and/or bubble formation. These deviations can give overestimation or underestimation of GPP (Cox et al. in prep.).
Back to top
How do TIDE estuaries function as a filter for nutrients?
What are the important factors controlling ecosystem functioning?
What is important in establishing a zonation for estuaries?
What parameters should be used to define and evaluate measure targets?
Which measures are suitable to improve the physical characteristics and chemical water quality?
Which variables limit estuarine primary production?
An interestuarine comparison for ecology in TIDE
Table of content
- 1. Introduction
- 1a. Biogeochemical processing for N, P and Si in Northern temperate estuaries
- 1b. Nutrient fluxes
- 1c. Primary production
- 1d. Differences and similarities between Elbe, Scheldt, Humber and Weser
- 2. Material and methods
- 2a. Study area & period
- 2b. Physics
- 2c. Biogeochemistry
- 3. Results
- 3a. Physics 2
- 3b. Biogeochemistry 2
- 4. Discussion
- 4a. Estuarine patterns
- 4b. Different sink and source functions for nutrients
- 4c. Oxygen deficiencies
- 4d. Primary production 2
- 5. Conclusion
- 5a. What are the important factors controlling ecosystem functioning within the TIDE estuaries?
- 5b. How do TIDE estuaries function as a filter for nutrients?
- 5c. How can we avoid oxygen deficiency situations in the TIDE estuaries?
- 5d. Which variables limit primary production in the TIDE estuaries?
- 5e. Lessons learnt from TIDE: towards ecosystem services and measures, recommendations for further estuarine management
- 6. References
Authors:
by Geerts L, Maris T, Meire P
With contributions of Beauchard O, Schöl A, Vandenbruwaene W., Van Damme S, Wolfstein K, Manson S, Saathoff S, Soetaert K, Cox T, Meire A, (February 2013)
by Geerts L, Maris T, Meire P
With contributions of Beauchard O, Schöl A, Vandenbruwaene W., Van Damme S, Wolfstein K, Manson S, Saathoff S, Soetaert K, Cox T, Meire A, (February 2013)
2c. Biogeochemistry
Estuarine ecosystems comprise the complex interaction between biotic and abiotic components. To understand the underlying processes that define water quality and ecology, regular biogeochemical (table 2) and continuous oxygen data have been gathered. To compare the data of the four estuaries they were classified into different zones related to chlorinity (Venice classification, see also Geerts et al. 2012 ). For further details on data availability per season and estuarine zone, see table 1 and Attachment 1.As is clear from this table, comparison for biogeochemistry for all four TIDE estuaries can only be applied on a limited set of parameters. Furthermore, not all zones are equally represented in every estuary (table 1). Comparison and analyses are performed whenever at least two estuaries have data for the parameters of interest for the research question addressed (see ‘1 Introduction’ ).
For summary statistics: number (n), mean, median, standard error, minimum and maximum of all variables
- in general for a whole year,
- in general for winter and summer season,
- & per estuarine zone according to the Venice-classification,
Table 2 Summary overview of biogeochemical data availability per estuary (x* very few data points, x deduced data, x(!) chlorophyll extract for the Humber estuary, chlorophyll a data not available)
| Parameter | Elbe | Scheldt | Humber | Weser |
| Chlorinity (CL) | x | x | x | x |
| Temperature (T) | x | x | x | x |
| pH | x | x | x | x |
| Conductivity (COND) | x | x | x | x |
| Dissolved oxygen (DO) | x | x | x | x |
| Dissolved oxygen saturation (DOsat) | x | x | x | x |
| Ammonium (NH4) | x | x | x | x |
| Nitrate (NO3) | x | x | x | x |
| Nitrite (NO2) | x | x | x | x |
| Organic nitrogen (ORG_N) | x | x | x * | x |
| Total dissolved inorganic nitrogen (TDIN) | x | x | x | x |
| Suspended particulate matter (SPM) | x | x | x | x |
| Chlorophyll a (CHL_A) | x | x | x (!) | x * |
| Phosphate (PO4) | x | x | x | x |
| Total phosphorus (TP) | x | x | x | |
| Dissolved silica (DSI) | x | x | x * | x * |
| Biological oxygen demand (BOD) | x | x | x | x |
| Phaeopigments (PHAE) | x | x | x * | |
| Dissolved organic carbon (DOC) | x | x | x | |
| Particulate organic carbon (POC) | x | x | x | |
| Total organic carbon (TOC) | x | x |
General parameters
Spatial and temporal distribution of temperature, pH, chlorinity and suspended particulate matter are discussed for each TIDE estuary. Surfer plots were made for suspended particulate matter concentrations for the Elbe, Scheldt and Humber estuary for the time period studied. Because of differences between sampling campaigns in the three estuaries, surfer plots were based upon seasonal (3 month) averages for each TIDE-km sampled. Spring corresponds to March, April and May. Summer includes June, July and August. Autumn comprises September, October, November and winter corresponds to December, January and February. For the Weser suspended particulate matter concentrations are represented as six-yearly averages on a yearly, summer and winter basis per sampling point.Furthermore, light climate is discussed by approximation of euphotic depth and mixing depth. Euphotic depth is calculated from suspended particulate matter concentrations with the following formulas according to Holzhauer et al. 2011:
- Kd = 0,053 SPM + 2,27
Zeuph = (-1/Kd) * ln(0,01) ~ 4,6/Kd
- Zmix = bathymetrical depth
Estuarine patterns
Correlation analyses
For all data gathered, correlation matrices were calculated within all four TIDE estuaries, wherefore Kendall’s tau was used to estimate a rank-based measure of association. This more robust method is used, because not all bivariate data is normally distributed. Association is only calculated when pairs (x, y) were complete. Correlation analyses allow us to examine estuarine patterns within each estuary to a maximum extent.Multivariate analyses
A more extensive and equilibrated examination of estuarine functioning per zone and season in different estuaries was performed using a multivariate analysis technique, principal component analysis. For this, data is necessary for all zones and seasons. Since for the Weser only data was available from 2004 to 2009 for mostly the freshwater and oligohaline zone, the Weser could not be further included in this analysis. Shared data between the Elbe, Scheldt and Humber include the following 13 variables: temperature, chlorinity, pH, suspended particulate matter, dissolved oxygen, dissolved oxygen saturation, nitrate, nitrite, total dissolved inorganic nitrogen, ammonium, phosphate, dissolved silica and chlorophyll a. Some missing chlorophyll a data in the Elbe estuary has been filled in using the linear relationship found with biological oxygen demand (R2 = 0.72). Missing dissolved silica data for the Humber estuary has been filled in using the linear relationship found with nitrate (R2 = 0.28). Chlorophyll data in the Humber represents chlorophyll extract and not chlorophyll a data. Nevertheless, after normalization and standardization the latter should also indicate chlorophyll‘s importance in the Humber estuary.Separate principal component analyses (PCA, Dolédec and Chessel 1987; Thioulouse et al. 2004) were performed for each estuary. Between-class analyses revealed the structuring influence for year, zone and season. The assessment involves a Monte-Carlo procedure based on 999 random permutations of the lines of the data table returning 999 simulated values of inertia, plus the observed one; the significance of the effect is then tested by the proportion of simulated values greater than the observed one (Heo and Gabriel 1999).
The partial triadic analysis (PTA, Thioulouse and Chessel 1987; Blanc et al. 1998; Thioulouse et al. 2004) is a sophisticated within-group analysis whereby the influence of a given factor (e.g. salinity zone or season here) is masked. In this multi-table approach, a set of k tables are seen as k clouds of points between which structural similarities are investigated. Its application here is especially relevant since the data form a cube defined by fully matched tables (e.g. same seasons × same descriptors × same zones). The columns of each table are initially centered (value minus mean value) so that all the clouds of point have a common geometric origin, and by this way, the considered factor is masked and the amplitude of each cloud of point is conserved. The procedure comprises two main steps:
(1) The construction of a correlation matrix summarizing the strength of multidimensional similarity between the tables; this step is called “interstructure”, and consists in identifying the most similar / deviant patterns by mean of a correlation circle; the statistics used is the Rv coefficient correlation (Robert and Escoufier 1976), and its significance is tested based on 999 random permutations of the lines of the tables.
(2) The construction of a system of axes encompassing the common processes between the tables as a common model, called “compromise”. As a second sub-step, the projection of the lines and columns of the different tables on the axes provides a mechanistic understanding of the similarities / deviations highlighted in the so-called “intrastructure”.
Nutrients
Time-distance surfer plots
Surfer plots were made for dissolved inorganic nutrients (total dissolved inorganic nitrogen, ammonium, nitrate, phosphate, dissolved silica) for the Elbe, Scheldt and Humber estuary for the time period studied. Because of differences between sampling campaigns in the three estuaries, surfer plots were based upon seasonal (3 month) averages for each TIDE-km sampled. Spring corresponds to March, April and May. Summer includes June, July and August. Autumn comprises September, October, November and winter corresponds to December, January and February. The Weser sampling campaign only comprises three consequent sampling points, of which two in the freshwater zone and one in the oligohaline zone. Therefore, it was not possible to include surfer plots for the latter estuary. For the Weser nutrient concentrations are represented as overall six-yearly, summer and winter averages per sampling point.Mixing plots
For each sampling point along the estuarine gradient the expected concentration by interpolation was calculated according to the conservative mixing theory as described in Eyre (2000). This is based on the chlorinity gradient and the fresh- and saltwater end member concentrations of the substrate (N, P, DSi or DO) considered. In TIDE the outer sea limit for the different estuaries studied has not always been defined up to 35 ‰. Therefore, the interpolation has been performed per set of three sampling points. Actually, chlorinity is used to calculate a weighed concentration by interpolation between each up- and downstream sampling point. Thus, formula (2) is deduced from the original formula (1) according to Eyre et al. (2000) (fig. 6). Next, to calculate the so-called ‘gain’ or ‘loss’ with respect to the expected concentration by interpolation, a difference is made between the observed and expected concentration. This was corrected for the distance between the sampling points and has been plotted as time-distance surfer plots. Green zones represent areas of gain, while red zones show areas of loss. The conservative mixing gives the concentration as expected when only dilution due to tidal mixing is observed. Hence, any deviation from these calculated concentrations, positive (gain) or negative (loss), could represent estuarine processes e.g. denitrification or direct input or removal, e.g. tributary input or freshwater discharge respectively.To have a more overall view, this gain or loss has also been multiplied with freshwater discharge, hence total gain or loss can be compared between estuaries. This has been performed for the whole estuarine gradient and per zone. Filter efficiencies have been calculated in percentage as the gain or loss according to the input concentration. The input concentrations per zone are defined as the most upstream concentration of that zone.
Residence time can reach up to about maximum 40 days in the freshwater zone (Elbe) and about maximum 50 days in the polyhaline zone (Scheldt) in summer. Thus, seasonal gain or loss can be considered. However, a yearly average can be considered more accurate, since transient effects are attenuated in this way.
Oxygen and primary production
Dissolved oxygen concentration and saturation
Surfer plots were made for dissolved oxygen concentrations for the Elbe, Scheldt and Humber estuary for the time period studied. Furthermore, dissolved oxygen saturation concentrations larger than 100% are indicated with diamonds. Because of differences between sampling campaigns in the three estuaries, surfer plots were based upon seasonal (3 month) averages for each TIDE-km sampled. Spring corresponds to March, April and May. Summer includes June, July and August. Autumn comprises September, October, November and winter corresponds to December, January and February. For the Weser dissolved oxygen concentrations and dissolved oxygen saturation concentrations are represented as six-yearly general, summer and winter average per sampling point.Oxygen, gain and loss
The conservative mixing principle is applied similarly as for the nutrient concentrations, described earlier (see “2.3.2.2 Mixing plots“). This method only works for dissolved constituents. Although oxygen is gaseous, it can be considered fully dissolved and thus behaving much in a similar way as transport of nutrients.Biological oxygen demand
Surfer plots were made for biological oxygen demand for the Elbe and Scheldt estuaries for the time period studied. In the Elbe the biological oxygen demand is measured over a period of 7 days. Within the boundary sampling station ‘Schnackenburg’, which is located upstream the weir and thus in the riverine part of the Elbe, biological oxygen demand has been measured for several time periods, of which also over 5 days and 7 days. The average seasonal ratio of ‘(biological oxygen demand over 5 days)/(biological oxygen demand over 7 days)’ for this station has been used to calculate the approximate biological oxygen demand over 5 days for the other sampling points, hence the Elbe biological oxygen demand can now be compared with the Scheldt estuary. Because of differences between sampling campaigns in the two estuaries, surfer plots were based upon seasonal averages per distance sampled. In both the Weser and Humber estuaries biological oxygen demand has not been measured.Chlorophyll a concentration
Surfer plots were made for chlorophyll a concentrations for the Elbe, Scheldt and for chlorophyll extract concentrations for the Humber estuary for the time period studied. Because of differences between sampling campaigns in the three estuaries, surfer plots were based upon seasonal (3 month) averages for each TIDE-km sampled. Spring corresponds to March, April and May. Summer includes June, July and August. Autumn comprises September, October, November and winter corresponds to December, January and February. For the Weser chlorophyll a concentrations were only measured at the boundary station Bremen Hemelingen and are therefore not represented.Gross primary production
Primary production is not linearly correlated to chlorophyll a concentrations. Often estimates of primary production are made based upon 14C-incorporation. However, this method is time-consuming, expensive and bottle-effects may underestimate true primary production. Using a Fourier transformation can decompose periodic components from continuous oxygen data series. Assuming diurnal patterns in oxygen time series are mainly effect of primary production, applying the Fourier-method as described in detail in Cox et al. (in prep.) allows estimation of gross primary production (GPP) (fig. 7).A truncated sinusoid (fig. 8) relation is applied between the time average and Fourier amplitude, which is function of the fraction of daylight. This transformation assumes a constant daylight fraction. Therefore, only time series of 14 days are considered at once.
The method is less applicable when the estuarine system is stratified, shallow, subject to short residence times, experiencing strong air-water exchange and/or bubble formation. These deviations can give overestimation or underestimation of GPP (Cox et al. in prep.).
Nutrient ratios
When measured, molar nutrient ratios of nitrogen-phosphate, nitrogen-dissolved silica and phosphate-dissolved silica were calculated and are represented as overall six-yearly, summer and winter averages per sampling point. Nitrogen within each ratio refers to the sum of dissolved inorganic nitrogen. Redfield ratios (C/N/P/Si~106/16/1/16) are displayed as well to detect potential nutrient limitations for diatoms. However, potential nutrient limitation does not necessarily imply actual nutrient limitation. E.g. the nutrient ratio for nitrogen to phosphorus could be lower than 16, indicating a potential limitation for nitrogen. Nonetheless, nitrogen concentration could be sufficient to sustain the diatom population, since other factors, e.g. light, could inhibit algal growth and not all nitrogen and phosphorus are used up to the limit.Important to know
Reports / Measures / Tools
| Report: | Zonation of the TIDE estuaries (Functioning) |
|---|---|
| Report: | Interestuarine comparison: Hydro-geomorphology (Functioning) |
Management issues
How can we avoid oxygen deficiency situations?How do TIDE estuaries function as a filter for nutrients?
What are the important factors controlling ecosystem functioning?
What is important in establishing a zonation for estuaries?
What parameters should be used to define and evaluate measure targets?
Which measures are suitable to improve the physical characteristics and chemical water quality?
Which variables limit estuarine primary production?